1） 个人简介
徐明周，主要从事概率论与数理统计方面的研究工作。我在博士期间的博士论文选题是次线性期望下的大偏差和扩散过程.我在博士期间的主要方向也是大偏差原理及其相关的概率极限理论.主要研究兴趣是与大偏差原理相关的各种概率极限理论和非线性期望、次线性期望相关理论.
2)大学开始受教育经历
2001年-2005年，武汉大学，数学与统计学院统计专业，学士，导师高付清教授
2005年-2011年，武汉大学，数学与统计学院概率论与数理统计专业，博士，导师高付清教授
2） 研究工作经历
2010年7月-2011年6月，江汉大学，数计学院，教师
2011年7月至今，景德镇陶瓷学院，信息工程学院，教师
3） 科研成果
高付清，徐明周，次线性期望下独立随机变量列的大偏差和中偏差，中国科学：数学2011年 第41卷 第4期：337～352
Fuqing Gao, Mingzhou Xu, The support of the solution for stochastic differential equations driven by G-Brownian motion, Acta Mathematica Sinica, English Series, 2012, Vol.28, No.12, 2417-2430.
Fuqing Gao, Mingzhou Xu, Relative entropy and Large deviations under sublinear expectations, Acta Mathematica Scientia, 2012, 32(B)5: 1826-1834.
Mingzhou Xu, Yongzheng Zhou, Large deviations for a test of symmetry based on kernel density estimator in
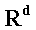,Chinese Journal of Applied Probability and Statistics, 2015,Vol.31, No.3, 238-246.
徐明周，周永正， 基于
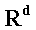上核密度估计的对称检验的中偏差，数学实践与认识2015年 23期
Mingzhou Xu, Yunzheng Ding,Yongzheng Zhou, Precise Rates in the Generalized Law of the Iterated Logarithm in R^m, Journal of Mathematical Research with Applications,2018, Vol. 38, No. 1, pp. 103-110.CSCD
Mingzhou Xu, Yunzheng Ding,Yongzheng Zhou,Central limit theorem and moderate deviation for nonhomogeneous Markov chains, J. of Math.(PRC),2019,Vol.39, No.1, 137-146
Mingzhou Xu,Yunzheng Ding,Yongzheng Zhou,Moderate deviations in $L_1(\rr^d)$ for a test of symmetry based on kernel density estimator,Chinese Journal of Applied Probability and Statistics, 2019,Vol.35, No.2, 141-152, CSCD
Mingzhou Xu, Kun Cheng, Yunzheng Ding, Youzheng Zhou. Probability inequalities and Rosenthal inequalities for the sequence of martingale differences, J.of Math.(PRC), 2019,Vol.39, No.5,105-712,
Mingzhou Xu, Kun Cheng, Yunzheng Ding,Moderate deviations for nonhomogeneous Markov chains,Statistics and Probability Letters, 157 (2020) 108632, ,SCI收入
等
联系方式：mingzhouxu@hotmail.com;
mingzhouxu@whu.edu.cn
徐明周主持或参与科研项目：
1. 江西省教育厅科研青年项目，核密度估计L_1空间下的中偏差和次线性空间下的大偏差，2016/01-2018/09，2万元，完成，主持
2. 江西省自然科学基金项目，基于广义Liu型估计的古陶瓷类文物鉴定研究，在研，参与
3. 江西省教育厅科研一般项目,核密度估计、非时齐马氏链等中偏差大偏差和极限定理,2020/01-2022/12，3万元，主持
